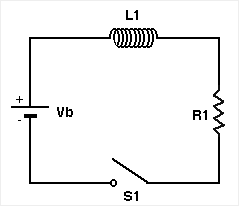
1.  Consider a circuit where a battery, an inductor and a resistor are connected in series, as shown below.  If the switch, S1, is closed at t0 =0 , the current in the inductor (circuit), at a later time, t, is given by

    I2 = If*(1 - exp(- t/t)))

where If = Vb/R is the current in the circuit for times very large compared to t and t = L/R , the LR time constant for the circuit.



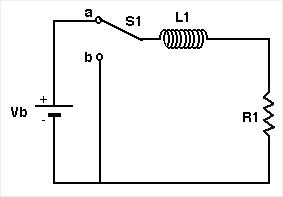
2.  Consider the circuit shown below where S1 has been in position a for a long time.  At t0=0 S1 is switched to position b, removing the battery from the circuit.  The current in the inductor (circuit) at a later time t is given by

    I2 = I0*exp(-t/t)

where I0 = Vb/R is the current through the inductor at t=0  and t and t = L/R , the LR time constant for the circuit.  Since the current through an inductor cannot change instantaneously, the current through the inductor just before S1 is moved from position a to position b must be the same as the current just after the switch moves from a to b.